Do you interested to find 'homework 4 1 combining functions answers'? All the details can be found here.
Table of contents
- Homework 4 1 combining functions answers in 2021
- Combining functions calculator two terms
- Solving functions
- Algebra 2
- Combining functions calculator
- Composition of functions worksheet answer key pdf
- Composite functions
- Function composition
Homework 4 1 combining functions answers in 2021
 This picture demonstrates homework 4 1 combining functions answers.
This picture demonstrates homework 4 1 combining functions answers.
Combining functions calculator two terms
 This image demonstrates Combining functions calculator two terms.
This image demonstrates Combining functions calculator two terms.
Solving functions
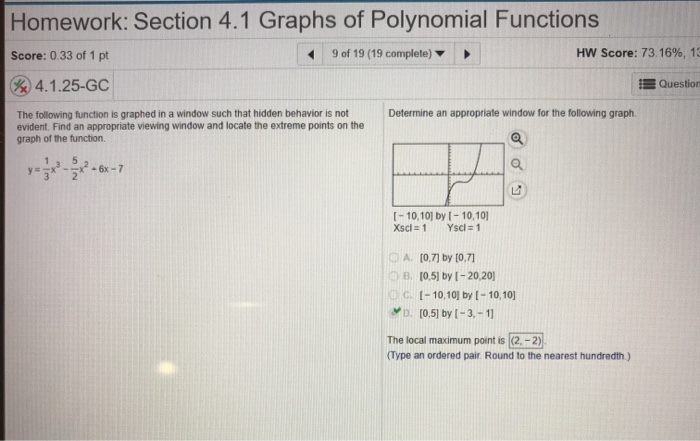 This picture illustrates Solving functions.
This picture illustrates Solving functions.
Algebra 2
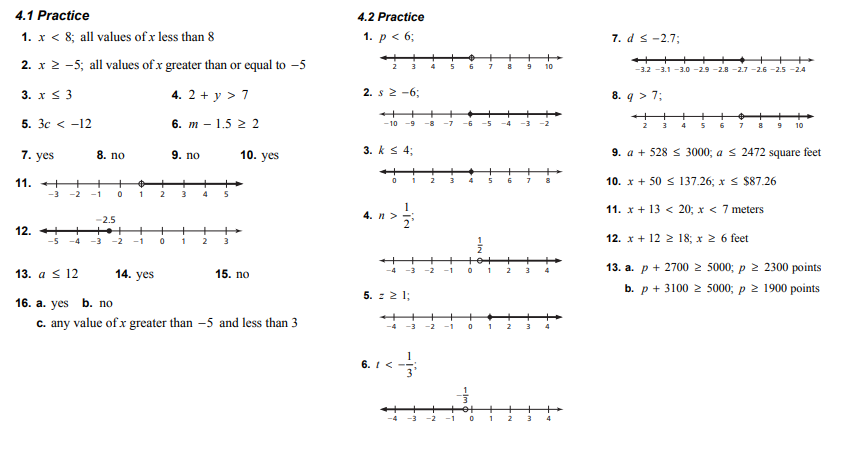 This picture representes Algebra 2.
This picture representes Algebra 2.
Combining functions calculator
 This picture illustrates Combining functions calculator.
This picture illustrates Combining functions calculator.
Composition of functions worksheet answer key pdf
 This image representes Composition of functions worksheet answer key pdf.
This image representes Composition of functions worksheet answer key pdf.
Composite functions
 This picture shows Composite functions.
This picture shows Composite functions.
Function composition
 This picture illustrates Function composition.
This picture illustrates Function composition.
How to find and simplify the composition of functions?
Find and simplify the functions (g − f)(x) and (g f)(x), given f(x) = x − 1 and g(x) = x2 − 1. Are they the same function? Begin by writing the general form, and then substitute the given functions. No, the functions are not the same.
When do you substitute a function for a given function?
Begin by writing the general form, and then substitute the given functions. No, the functions are not the same. Note: For (g f)(x), the condition x ≠ 1 is necessary because when x = 1, the denominator is equal to 0, which makes the function undefined.
Which is an example of a combining function?
Example 2 Given f (x) = 2 +3x−x2 f ( x) = 2 + 3 x − x 2 and g(x) = 2x −1 g ( x) = 2 x − 1 evaluate each of the following. These are the same functions that we used in the first set of examples and we’ve already done this part there so we won’t redo all the work here.
When do we call an operation a composition of functions?
When the output of one function is used as the input of another, we call the entire operation a composition of functions. For any input x and functions f and g, this action defines a composite function, which we write as f ∘ g such that (f ∘ g)(x) = f(g(x))
Last Update: Oct 2021